GeoDIB

Nov 1, 2006
website
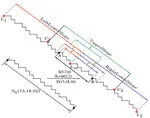
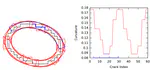
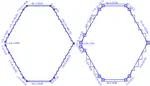
Heterogenous data

This project gathered members of four laboratories: LORIA (Leader, Nancy), LIRIS (Lyon), LAIC (Clermont), LaBRI (Bordeaux) then LAMA (Chambéry).

digital geometry noisy geometry arithmetic 2D 3D digital straightness digital planarity
Jacques-Olivier Lachaud
Jacques-Olivier Lachaud
Professor of Computer Science

My research interests include digital geometry, geometry processing, image analysis, variational models and discrete calculus.

Publications

Analytical description of digital intersections: Minimal parameters and multiscale representation
The paper contributes to a multiscale theory of digital shapes by presenting novel methods for a multiscale representation of digital …
Mouhammad Said, Jacques-Olivier Lachaud
PDF Cite Project
Analytical description of digital intersections: Minimal parameters and multiscale representation
Extraction of Connected Region Boundary in Multidimensional Images
This paper presents an algorithm to extract the boundary of a connected region(s) using classical topology definitions. From a given …
D. Coeurjolly, B. Kerautret, Jacques-Olivier Lachaud
PDF Cite Project DOI IPOL demo URL
Extraction of Connected Region Boundary in Multidimensional Images
Meaningful Scales Detection: an Unsupervised Noise Detection Algorithm for Digital Contours
This work presents an algorithm which permits to detect locally on digital contour what is the amount of noise estimated from a given …
B. Kerautret, Jacques-Olivier Lachaud
PDF Cite Project DOI IPOL demo URL
Meaningful Scales Detection: an Unsupervised Noise Detection Algorithm for Digital Contours
Two efficient algorithms for computing the characteristics of a subsegment of a digital straight line
We address the problem of computing the exact characteristics of any subsegment of a Digital Straight Line (DSL) with known …
Jacques-Olivier Lachaud, M. Said
PDF Cite Project Project DOI Code (DGtal) URL
Two efficient algorithms for computing the characteristics of a subsegment of a digital straight line
Digital Shape Analysis with Maximal Segments
We show in this paper how a digital shape can be efficiently analyzed through the maximal segments defined along its digital contour. …
Jacques-Olivier Lachaud
PDF Cite Project URL
Digital Shape Analysis with Maximal Segments
Meaningful Scales Detection along Digital Contours for Unsupervised Local Noise Estimation
The automatic detection of noisy or damaged parts along digital contours is a difficult problem since it is hard to distinguish between …
B. Kerautret, Jacques-Olivier Lachaud
PDF Cite Project DOI IPOL demo
Meaningful Scales Detection along Digital Contours for Unsupervised Local Noise Estimation
Meaningful Thickness Detection On Polygonal Curve
The notion of meaningful scale was recently introduced to detect the amount of noise present along a digital contour (Kerautret and …
B. Kerautret, Jacques-Olivier Lachaud, M. Said
PDF Cite Project Slides (PDF)
Meaningful Thickness Detection On Polygonal Curve
Circular arc reconstruction of digital contours with chosen Hausdorff error
We address the problem of constructing an approximate continuous representation of a digital contour with guarantees on the Hausdorff …
B. Kerautret, Jacques-Olivier Lachaud, T. P. Nguyen
PDF Cite Project Poster (PDF)
Circular arc reconstruction of digital contours with chosen Hausdorff error
Computing the Characteristics of a SubSegment of a Digital Straight Line in Logarithmic Time
We address the problem of computing the exact characteristics of any subsegment of a digital straight line with known characteristics. …
M. Said, Jacques-Olivier Lachaud
PDF Cite Project Code (DGtal)
Computing the Characteristics of a SubSegment of a Digital Straight Line in Logarithmic Time
Delaunay properties of digital straight segments
We present new results concerning the Delaunay triangulation of the set of points of pieces of digital straight lines. More precisely, …
T. Roussillon, Jacques-Olivier Lachaud
PDF Cite Project
Delaunay properties of digital straight segments
Accurate Curvature Estimation along Digital Contours with Maximal Digital Circular Arcs
We propose in this paper a new curvature estimator based on the set of maximal digital circular arcs. For strictly convex shapes with …
T Roussillon, Jacques-Olivier Lachaud
PDF Cite Project
Accurate Curvature Estimation along Digital Contours with Maximal Digital Circular Arcs
Dynamic Minimum Length Polygon
This paper presents a formal framework for representing all reversible polygonalizations of a digital contour (i.e. the boundary of a …
Jacques-Olivier Lachaud, X. Provençal
PDF Cite Project Slides (PDF)
Dynamic Minimum Length Polygon
Maximal Planes and Multiscale Tangential Cover of 3D Digital Objects
The sequence of maximal segments (i.e. the tangential cover) along a digital boundary is an essential tool for analyzing the geometry …
E. Charrier, Jacques-Olivier Lachaud
PDF Cite Project
Maximal Planes and Multiscale Tangential Cover of 3D Digital Objects
Unsupervised, Fast and Precise Recognition of Digital Arcs in Noisy Images
In image processing and pattern recognition, the accuracy of most algorithms is dependent on a good parameterization, generally a …
T. P. Nguyen, B. Kerautret, I. Debled-Rennesson, Jacques-Olivier Lachaud
PDF Cite Project
Unsupervised, Fast and Precise Recognition of Digital Arcs in Noisy Images
Digital shape analysis with maximal segments
It is often interesting to study the geometry of digitization of euclidean shapes in the plane, and to establish connections between …
Jacques-Olivier Lachaud
PDF Cite Project
Digital shape analysis with maximal segments
Curvature estimation along noisy digital contours by approximate global optimization
In this paper, we introduce a new curvature estimator along digital contours, which we called global min-curvature (GMC) estimator. As …
B. Kerautret, Jacques-Olivier Lachaud
PDF Cite Project DOI URL
Curvature estimation along noisy digital contours by approximate global optimization
Multiscale Analysis of Discrete Contours for Unsupervised Noise Detection
Blurred segments [2] were introduced in discrete geometry to address possible noise along discrete contours. The noise is not really …
B. Kerautret, Jacques-Olivier Lachaud
PDF Cite Project URL
Multiscale Analysis of Discrete Contours for Unsupervised Noise Detection
Multiscale Discrete Geometry
This paper presents a first step in analyzing how digital shapes behave with respect to multiresolution. We first present an analysis …
M. Said, Jacques-Olivier Lachaud, F. Feschet
PDF Cite Project URL
Multiscale Discrete Geometry
Comparison of Discrete Curvature Estimators and Application to Corner Detection
Several curvature estimators along digital contours were proposed in recent works [1,2,3]. These estimators are adapted to non perfect …
B. Kerautret, Jacques-Olivier Lachaud, B. Naegel
PDF Cite Project
Comparison of Discrete Curvature Estimators and Application to Corner Detection
Discrete Contour Extraction from Reference Curvature Function
A robust discrete curvature estimator was recently proposed by Kerautret et al. [1]. In this paper, we exploit the precision and …
H. G. Nguyen, B. Kerautret, P. Desbarats, Jacques-Olivier Lachaud
PDF Cite Project Poster (PDF)
Discrete Contour Extraction from Reference Curvature Function
Robust estimation of curvature along digital contours with global optimization
In this paper we introduce a new curvature estimator based on global optimisation. This method called Global Min-Curvature exploits the …
B. Kerautret, Jacques-Olivier Lachaud
PDF Cite Project Poster (PDF)
Robust estimation of curvature along digital contours with global optimization
Curvature based corner detector for discrete, noisy and multi-scale contours
Estimating curvature on digital shapes is known to be a difficult problem even in high resolution images. Moreover the presence of …
B. Kerautret, Jacques-Olivier Lachaud, B. Naegel
PDF Cite Project DOI
Curvature based corner detector for discrete, noisy and multi-scale contours

Events

Multigrid convergence of digital curvature estimators
Invited talk.
Nov 18, 2013 12:00 AM — Nov 22, 2013 12:00 AM Centre International de Recherche Mathématique (CIRM), Luminy, France
Jacques-Olivier Lachaud
Code Project Project Publication Slides (PDF) Paper (PDF) URL
Multigrid convergence of digital curvature estimators
Asymptotic linear digital geometry
Invited talk.
Feb 8, 2011 12:00 AM — 12:00 AM Paris, France
Jacques-Olivier Lachaud
Project Slides (PDF)
Asymptotic linear digital geometry
Asymptotic linear digital geometry and applications
Invited talk.
Feb 8, 2011 12:00 AM — 12:00 AM Perpignan, France
Jacques-Olivier Lachaud
Project Slides (PDF)
Asymptotic linear digital geometry and applications