Discrete calculus model of Ambrosio-Tortorelli’s functional

Abstract

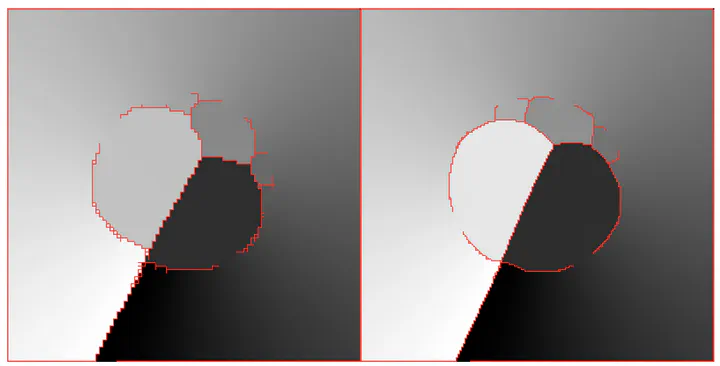
Ambrosio-Tortorelli’s functional (AT) is a well known relaxation of the classical Mumford-Shah model (MS). AT involves both a reconstruction function u and an approximation of the set of discontinuities v in its formulation. AT has the nice property to Gamma-converge toward MS while being much simpler to solve. However its numerical approximation suffers from a technical difficulty: it is difficult to make the set of discontinuities thin at any digitisation scale, thus making the numerical result poor around discontinuities. We propose a discrete calculus model of AT, whose formulation authorises thin discontinuities at the scale of interest. We will recall the main aspects of discrete calculus, and present our discrete AT model. We will then show that this formulation is versatile enough to address several problems of image and geometry processing: image restoration, segmentation and inpainting, digital surface normal field regularisation, or geometric mesh denoising, inpainting or segmentation.

Jacques-Olivier Lachaud

Professor of Computer Science

My research interests include digital geometry, geometry processing, image analysis, variational models and discrete calculus.