Discrete deformable boundaries for the segmentation of multidimensional images

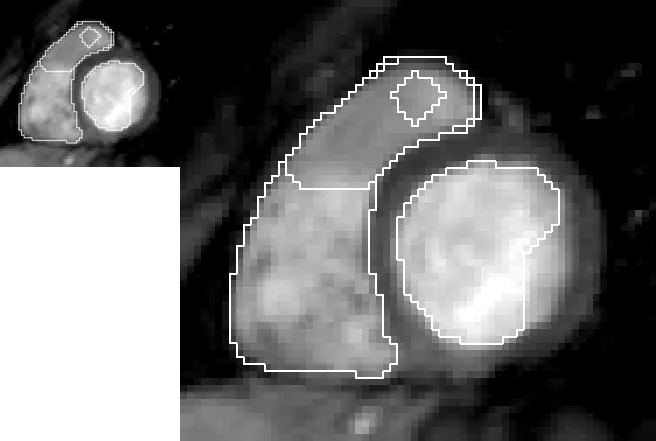
Energy-minimizing techniques are an interesting approach to the segmentation problem. They extract image components by deforming a geometric model according to energy constraints. This paper pro­poses an extension to these works, which can segment arbitrarily complex image components in any dimension. The geometric model is a digital surface with which an energy is associated. The model grows inside the component to segment by following minimal energy paths. The segmentation result is obtained em a posteriori by examining the energies of the successive model shapes. We validate our approach on several 2D images.

Proc. 4th Int. Workshop on Visual Form (IWVF4), Capri, Italy, volume 2059 of Lecture Notes in Computer Science, pp 542-551, 2001. Springer